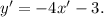Question

I need help with my math. I need help with 6.

Answer 1

Step-by-step explanation

We have the following information:

`(-2,5);y=-4x+2,`

and our task is to find the slope-intercept equation of the line that passes through the given point and is parallel to the graph of the given line.

Recall that the slope-intercept equation of any line has the following "framework":

`y^(\prime)=m\cdot x^(\prime)+b,_{}`

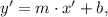
where "m" and "b" are constants called the slope of the line and the y-intercept, respectively. We want our line to be parallel to the graph of the given one; this means that the slope of both lines must be the same. Then,

`m=-4.`

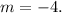
Then, our equation becomes

`y^(\prime)=-4x^(\prime)+b\text{.}`

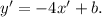
Now, we want the point (-2,5) to lie on the line. In other words, this point must "satisfy" our equation in the following sense:

`5=-4(-2)+b\text{.}`

Solving this equation for b, we get

`\begin{gathered} 5=-4(-2)+b, \\ 5=8+b, \\ 8+b=5, \\ -8+(8+b)=-8+5, \\ -8+8+b=-3, \\ b=-3. \end{gathered}`

Then, our desired equation is

`y^(\prime)=-4x^(\prime)-3.`

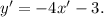
Graphically, all looks like

(The green line represents the given one, and the blue line represents the requested line. And clearly, the orange point is the given point)

Answer

The slope-intercept equation of the line that passes through the given point and is parallel to the graph of the given line is

`y^(\prime)=-4x^(\prime)-3.`