Answer:
The angle between the vector and positive x-axis is approximately 59.036º.
Step-by-step explanation:
By Linear Algebra and to be precise, by definition of Dot Product we can determine the angle between two vector from following expression:
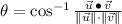 (1)
(1)
Where:
 ,
,
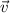 - Vectors, no unit.
- Vectors, no unit.
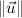 ,
,
 - Norms of vectors, no unit.
- Norms of vectors, no unit.
 - Angle, measured in sexagesimal degrees.
- Angle, measured in sexagesimal degrees.
Please notice that norms are calculated by Pythagorean Theorem. If we know that
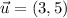 and
and
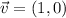 , then the angle between the vector and positive x-axis is:
, then the angle between the vector and positive x-axis is:

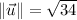


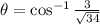
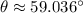
The angle between the vector and positive x-axis is approximately 59.036º.