Question

The solid below is dilated by a scale factor of 1/2. Find the surface area of the solid created upon dilation. Answer in terms of π

Answer 1

42pi square units

Step-by-step explanation

Given:

1. A cylinder dilated by a scale factor of 1/2

2. Radius = 7

3. Height = 5

Desired Outcome:

The surface area of the solid created upon dilation

Formula for Surface Area of a cylinder

`SA\text{ = 2}\pi rh+2\pi r^2`

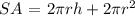
Surface of the cylinder before dilation

`\begin{gathered} SA\text{ \lparen before dilation\rparen = 2}\pi\cdot7\cdot5\text{ + 2}\pi\cdot7^2 \\ \text{ = 70}\pi\text{ + 98}\pi \\ \text{ = 168}\pi \end{gathered}`

Surface of the cylinder after dilation

`\begin{gathered} SA\text{ \lparen after dilation\rparen = 168}\pi*((1)/(2))^2 \\ \text{ = 168}\pi*(1)/(4) \\ \text{ = 42}\pi \end{gathered}`

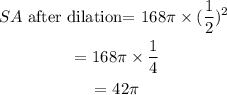
Hence, the surface area of the solid created upon dilation is 42pi square units.