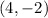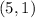Question

Which ordered pairs are solutions to the inequality y−3x<−4?

Select each correct answer.

(4, −2)

(0, −3)

(5, 1)

(−3, 0)

(1, −1)

Answer 1

(4,-2),(5,1)Are correct

Answer 2

we will proceed to verify each case to determine the solution of the problem

we know that

If the ordered pair is a solution of the inequality , then the ordered pair must be satisfied the inequality

we have

`y-3x < -4`

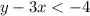
case A point
`(4,-2)`

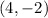
Substitute the values of x and y in the inequality

`-2-3*4 < -4`

`-14 < -4` -------> is true

therefore

the pair ordered
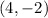`(4,-2)` is a solution of the inequality

case B point
`(0,-3)`

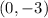
Substitute the values of x and y in the inequality

`-3-3*0 < -4`

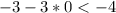
`-3 < -4` -------> is false

therefore

the pair ordered
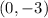`(0,-3)` is not a solution of the inequality

case C point
`(5,1)`

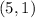
Substitute the values of x and y in the inequality

`1-3*5 < -4`

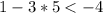
`-14 < -4` -------> is true

therefore

the pair ordered
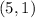`(5,1)` is a solution of the inequality

case D point
`(-3,0)`

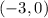
Substitute the values of x and y in the inequality

`0-3*(-3) < -4`

`9 < -4` -------> is false

therefore

the pair ordered
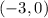`(-3,0)` is not a solution of the inequality

case E point
`(1,-1)`

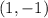
Substitute the values of x and y in the inequality

`-1-3*(1) < -4`

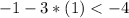
`-4 < -4` -------> is false

therefore

the pair ordered
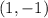`(1,-1)` is not a solution of the inequality

therefore

the answer is

`(4,-2)`

`(5,1)`