What is the most precise name for quadrilateral ABCD with vertices A(-4, -4), B(-4, -2), C(-1, -2), and D(-1, -4)? A) quadrilateral B) parallelogram C) rhombus D) rectangle

Question

asked Aug 26, 2017 225k views

2 Answers

Callo · Answer 1 · 2017-09-01T19:22:33+0000

Answer:

Option D.

Explanation:

The given vertices of quadrilateral ABCD are A(-4, -4), B(-4, -2), C(-1, -2), and D(-1, -4).

Distance formula:

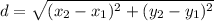
d=√((x_2-x_1)^2+(y_2-y_1)^2)

Using distance formula, we get

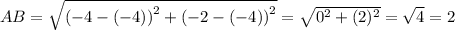
$AB=√(\left(-4-\left(-4\right)\right)^2+\left(-2-\left(-4\right)\right)^2)=√(0^2+(2)^2)=√(4)=2$

Similarly,

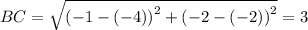
$BC=√(\left(-1-\left(-4\right)\right)^2+\left(-2-\left(-2\right)\right)^2)=3$

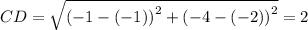
$CD=√(\left(-1-\left(-1\right)\right)^2+\left(-4-\left(-2\right)\right)^2)=2$

$AD=√(\left(-1-\left(-4\right)\right)^2+\left(-4-\left(-4\right)\right)^2)=3$

AB = CD

BC = AD

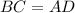
Measure of diagonals:

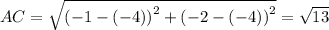
$AC=√(\left(-1-\left(-4\right)\right)^2+\left(-2-\left(-4\right)\right)^2)=√(13)$

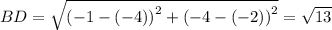
$BD=√(\left(-1-\left(-4\right)\right)^2+\left(-4-\left(-2\right)\right)^2)=√(13)$

AC = BD

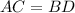
Since measure of opposite sides are equal and measure of diagonals are equal, therefore the given quadrilateral ABCD is a rectangle.

Hence, the correct option is D.