in a 130.0 g sample of tetraborane (B4H10), there are approximately 9.752 × 10²³ boron atoms.
Boron (B) atomic mass ≈ 10.81 g/mol
Hydrogen (H) atomic mass ≈ 1.01 g/mol
Molar mass of tetraborane (
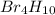 ) = (4 × Boron atomic mass) + (10 × Hydrogen atomic mass)
) = (4 × Boron atomic mass) + (10 × Hydrogen atomic mass)
Molar mass of tetraborane = (4 × 10.81 g/mol) + (10 × 1.01 g/mol)
Molar mass of tetraborane ≈ 43.24 g/mol + 10.1 g/mol
Molar mass of tetraborane ≈ 53.34 g/mol
Calculate the number of moles of tetraborane in 130.0 g:
Number of moles = Mass / Molar mass
Number of moles = 130.0 g / 53.34 g/mol
Number of moles ≈ 2.438 moles
Use the ratio of boron atoms per molecule of tetraborane:
From the chemical formula B4H10, there are 4 boron atoms per molecule of tetraborane.
Number of boron atoms = Number of moles of tetraborane × Number of boron atoms per mole
Number of boron atoms = 2.438 moles × 4
Number of boron atoms ≈ 9.752
Therefore, in a 130.0 g sample of tetraborane (B4H10), there are approximately 9.752 × 10²³ boron atoms.