Question

Priya drew a circle whose area is 25 cm. Dan drew a circle whose diameter is 4 times the radius of Priya's circle. How many times greater is the area of Dan's

circle?

Answer 1

400

Explanation:

first work out the radius of priya's circle with the equation a =
`\pi r^(2)`

`25 = \pi r^(2)`

`(25)/(\pi ) = r^(2)`

`r = \sqrt{(25)/(\pi ) }`

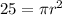
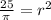
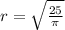
we know the diameter of a circle is 2 times the radius so the diameter of priya's circle is 2x
`\sqrt{(25)/(\pi ) }`

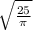
this means that if the diameter of dan's circle is 4 times bigger it must be

8x
`\sqrt{(25)/(\pi ) }`

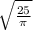
to find the radius of dan's circle we divide the diameter by to 2 to give

4x
`\sqrt{(25)/(\pi ) }`

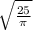
then use the a=
`\pi r^(2)` equation again

`a= (4 \sqrt{(25)/(\pi ) })^(2) \pi`

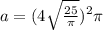
which equals 400

hope that helped