73 people
Step-by-step explanation
Step 1
the reception hall charges a $80 cleanup lee plus $33 per person
let x represents the number of invited people,
Let C represents the total cost,so
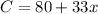
Now, if the budget is $2500, the cost must be equal or smaller than C, hence
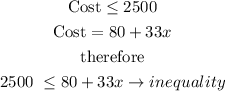
Step 2
now, solve the inequality

it means the greatest number of people Steve and MIchelle can invite is 73 people.
I hope this helps you