Question

3 support beams for a bridge form a pair of complementary angles. find the measure of each angle.

Answer 1

By definition, Complementary angles are those angles that add up to 90 degrees.

According to the information given in the exercise, the angles formed are Complementary angles. Then, you can set up the following equation:

`(4x)+(3x+13)=90`

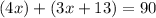
Now you must solve for the variable "x". This is:

`\begin{gathered} 4x+3x+13=90 \\ 7x=90-13 \\ 7x=77 \\ \\ x=(77)/(7) \\ \\ x=11 \end{gathered}`

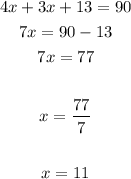
Knowing that the expressions that represent the angles are:

`\begin{gathered} (4x)\degree \\ \\ (3x+13)\degree \end{gathered}`

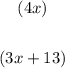
You can substitute the value of "x" into each expression and then evaluate, in order to find the measures of the angles. These are:

`\begin{gathered} (4\cdot11)\degree=44\degree \\ \\ (3\cdot11+13)\degree=46\degree \end{gathered}`

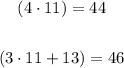
The answer is:

- Smaller angle:

`44\degree`

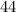
- Larger angle:

`46\degree`