Question

Which answer choice is equivalent to the expression below?

3x2y4/2xy
The 2 and 4 in the first part of problem(not the 2 from 2xy) are exponents.

A.3xy3/2 ( The second 3 is exponent )
B.3x3y5/2 (The second 3 is exponent, and the 5)
C.3/2xy3 ( The 3 in 2xy3 is exponent)
D.3xy2/2 ( The 2 in 3xy2 is an exponent.)

Answer 1

The correct option is A.

Explanation:

The given expression is

`(3x^2y^4)/(2xy)`

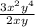
It can be written as

`(3)/(2)\cdot (x^2)/(x)\cdot (y^4)/(y)`

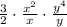
According to quotient property of exponent:

`(a^m)/(a^n)=a^(m-n)`

Using quotient property of exponent, we get

`(3)/(2)\cdot x^(2-1)\cdot y^(4-1)`

`(3)/(2)\cdot x\cdot y^(3)`

`(3xy^3)/(2)`

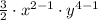
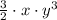
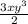
The expression
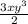`(3xy^3)/(2)` is equivalent to the given expression.

Therefore the correct option is A.

Answer 2

In order to know the equivalent expression of the given above, all we need to do is to simplify it. So, for the exponents, what we need to do is just subtract.
The final answer would be 3/2xy^3. So the answer is option C. Hope this is the answer that you are looking for.