Question

Convert the complex number 3 - 3i into its polar representation. a. 3(cos(60degrees) + isin(60degrees)) b. 3√2(cos(135degrees) + isin(135degrees)) c. √3(cos(225degrees) + isin(225degrees)) d. 3√(cos(315degrees) + isin(315degrees))

Answer 1

3sqrt(cos(315⁰)+isin(315⁰))

Explanation:

Answer 2

The correct option is B. 3√2(cos(135°) + i·sin(135°))

Explanation:

The complex number is given as : 3 - 3i

Now, comparing this with the standard for : a + bi

⇒ a = 3 and b = -3

Now, r = √a² + b²

⇒ r = √18

⇒ r = 3√2

`\tan\theta=(b)/(a)\\\\\implies\tan\theta=(-3)/(3)\\\\ \implies\tan\theta =-1\\\\ \implies\theta=(3\pi)/(4)\\\\\implies\theta=135`

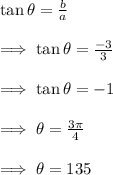
Now, The polar form is represented by :

z = r(cosθ + i·sinθ)

⇒ z = 3√2(cos 135° + i·sin 135°)

Therefore, the correct option is B. 3√2(cos(135°) + i·sin(135°))