Question

The bryant family reuion and the jordan family reunion both include a visit to the zoo desert archers different amounts for children and adults in the following system A represents the cost of an adult ticket and C represents the cost of a child ticket. The first equation in the system represents the amount of money the Bryant family spends and the second equation represents the amount of money that the Jordan family spends

12a + 16c =176
10a + 8c =120

Which of the following statements are true?

A. the Bryants take 12 adults and 16 children to the zoo

B. There is a total of 22 children at the reuions

C.There is a total of 18 people at the Jordans reuion

D.16 represents the cost of a child ticket for the Bryant reuion

E. 10 represents the cost of an adult ticket for the Jordan family

F. The Bryant family spends $120 on tickets for the children and adults

G. Together the family spends $296


❗PLEASE HELP❗

Answer 1

A,C,G statement is correct...

Answer 2

D. 16 represents the cost of a child ticket for the Bryant reunion.

G. Together the family spends $296

Explanation:

To find the right answer we have to solve the system of equation:

`\left \{ {{12a+16c=176} \atop {10a+8c=120}} \right.`

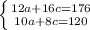
If we multiply the second equation by -2, we could eliminate the c-variable and solve for a-variable:

`\left \{ {{12a+16c=176} \atop {-20a-16c=-240}} \right.\\-8a=-64\\a=(-64)/(-8)=8`

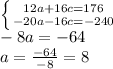
Now, we replace this value in one equation to obtain the other value:

`10a + 8c =120\\10(8)+8c=120\\80+8c=120\\8c=120-80\\c=(40)/(8)=5`

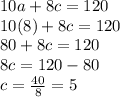
So, the solution of the system is
`(8,5)`, which means that there are 8 adults and 5 children.

Also, according to the linear expressions The Bryants spend $12 per adult and $16 per child, and a total amount of $176. The Jordan family spend $10 per adult and $8 per child, and a total amount of $120. Both families together spent $296.

Therefore, the right answers are D and G