Question

Based on the graph shown, which of the following statements is true?

median < mode
median = mode
median > mode

Answer 1

the median(300) = mode(300)

Answer 2

Option 2 is correct.

Explanation:

In the given graph x-axis represents the numbers and y-axis represent the frequency.

Number Frequency Cumulative frequency

100 14 14

200 6 20 (20<36)

300 18 38 (38>36)

400 12 50

500 2 52

600 12 64

700 8 72

Total 72

Mode is the number which has highest frequency. From the above table it is noticed that the highest frequency is 18 at 300. Therefore mode of the data is 300.

Sum of frequency is 72, which is an even number.

`Median=(n)/(2)\text{th term}`

`Median=(72)/(2)\text{th term}`

`Median=36\text{th term}`

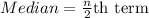
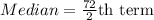
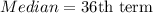
We have to find the number whose cumulative frequency is more than 36 but preceding cumulative frequency is less than 36.

Median of the graph is 300.

Since the value of median and mode are same, therefore

`median=mode`

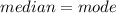
Option 2 is correct.