Answer:
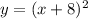
Explanation:
we have that
The ordered pairs
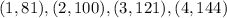 , and
, and
 represent a function
represent a function
The rule that that represent this function is equal to
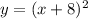
Verify
For
 -----> Find the value of y in the equation
-----> Find the value of y in the equation
 -----> is correct
-----> is correct
For
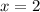 -----> Find the value of y in the equation
-----> Find the value of y in the equation
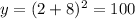 -----> is correct
-----> is correct
For
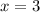 -----> Find the value of y in the equation
-----> Find the value of y in the equation
 -----> is correct
-----> is correct
For
 -----> Find the value of y in the equation
-----> Find the value of y in the equation
 -----> is correct
-----> is correct
For
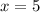 -----> Find the value of y in the equation
-----> Find the value of y in the equation
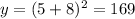 -----> is correct
-----> is correct