Answer:
The frequency is
 Hz
Hz
Explanation:
Sinusoid Function
The cosine and sine functions are generally called sinusoids because of their characteristic oscillatory behavior.
The general cosine function is expressed as
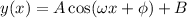
Where A is the amplitude, ω is the angular frequency, Ф is the phase shift and B is the midline or vertical shift.
We are given the function:

The coefficient of x is the angular frequency, thus

Since
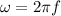
Where f is the frequency, then
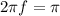
Simplifying:

The frequency is
 Hz
Hz