Answer:
In 3 hour 45 minutes Both working together do all of yard work.
Explanation:
Time taken by Steve to do yard work = 6 hours
Work Done by Steve in a hour =
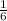
Time taken by Bill to do yard work = 10 hours
Work Done by Steve in a hour =
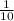
Work done by both in a hour =
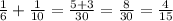
Time taken by by both working together =
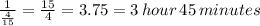
Therefore, In 3 hour 45 minutes Both working together do all of yard work.