Answer:
8 days
Explanation:
Direct Variation takes the form
 , and
, and
Inverse Variation takes the form

Where
 and
and
 are the 2 variables associated, and
are the 2 variables associated, and
 is the proportionality constant
is the proportionality constant
From the statement of the problem given, and taking time as
 and length as
and length as
 and number of men working as
and number of men working as
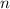
We can write:

Using the values given in the problem (
 ,
,
 , and
, and
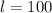 ), we can solve for k:
), we can solve for k:
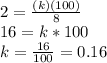
Now, we want to know time given
 and
and
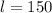 and
and

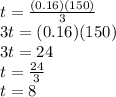
So, it will take 8 days.