Given the function
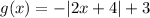
The expressions are equivalent if they show the same result as the original one.
A.
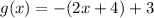
Since the terms held by the | | are positive, one solving the multiplication by -1 they will be negative with or without the module. This means that this expression is equivalent. (Alsmos the same just changed the module lines by parenthesis)
B.

C.
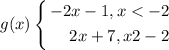
D.
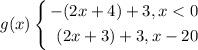
For the expressions that you posted, only A is equivalent.