If you are given a parabolic function you can algebraically determine the type of zeros of the function with the discriminant, which is
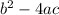
.
The discriminant is derived from the quadratic formula,
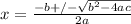
.
The discriminant is what's inside the square root (the radicand).
If the radicand is negative, there will be 2 imaginary numbers (there are two because of the plus or minus before the square root)
If the radicand is positive, there will be 2 real numbers.
If the radicand is zero, the answer will be 0.
Therefore,
If discriminant is negative, there are 2 complex zeros.
If discriminant is positive, there are 2 real zeros.
If discriminant is zero, there is one real zero.
A zero is basically the x-intercept.