Question

Will, Micah and sue went to dinner. Will paid 1/3 of the dinner bill. Micah and sue paid in the ratio of 2:5. If sue paid $6 more Than Will, how much did the dinner cost

Answer 1

$21. If you take 2:5 and double it 4:10, 10 is 6 more than four, so you add the two up (14) and then you have 2/3s of the bill. then you divide 14 in two (7) and multiply 7 by three (21).

Answer 2

Answer : The dinner cost was, $ 42

Step-by-step explanation :

Let the dinner cost be, 'x'

Dinner cost paid by Will =
`(1)/(3)* x`

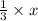
Remaining dinner cost =
`(1-(1)/(3))x=(2)/(3)x`

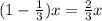
The ratio of dinner cost paid by Micah and sue is 2:5

Dinner cost paid by Micah =
`((2)/(3)x)* (2)/(7)=(4)/(21)x`

Dinner cost paid by Sue =
`((2)/(3)x)* (5)/(7)=(10)/(21)x`

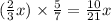
As, Sue paid dinner cost $6 more than Will.

That means,

`((10)/(21)x)=\$ 6+((1)/(3)* x)`

`((10)/(21)x)-((1)/(3)* x)=\$ 6`

`((1)/(7)* x)=\$ 6`

`x=\$ 6* 7`

`x=\$ 42`

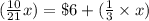
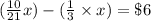
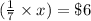
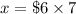
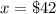
Thus, the dinner cost was, $ 42