Question

The ages of people who attend a local church are normally distributed. The mean age is 39.5, and the standard deviation is 18. Find the probability that a person chosen at random from this church is ages 21.5 or younger.

Answer 1

15.9%.

Step-by-step explanation:

The mean = 39.5

Standard deviation = 18

Raw Value, X=21.5

To find the probability that a person chosen at random from this church is ages 21.5 or younger, we first find the Z-Score.

`\begin{gathered} Z-\text{Score}=(X-\mu)/(\sigma) \\ =(21.5-39.5)/(18) \\ =(-18)/(18) \\ =-1 \end{gathered}`

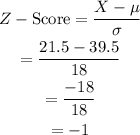
Next, we check the P-Value from the Z-Table.

`P(x<21.5)=0.15866`

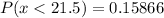
Therefore, the probability that a person chosen at random from this church is ages 21.5 or younger is 15.9%.