Question

Find the slope-intercept form of the equation of the line that is parallel to the line 6x - 3y = 5 and passes through the point (-2, 3)Show all work

Answer 1

Given the line parallel to the required equation of a line ase are asked tof

`6x-3y=5`

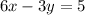
and the point the required line passes through as

`(-2,3)`

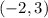
We can find the equation of the line parallel to 6x-3y=5 and passes through the point (-2,3) below:

Step-by-step explanation

First, we get the slope of the given line

The general equation of a line(slope -intercept form) is given as

`\begin{gathered} y=mx+c \\ We\text{ would rearrange 6x-3y=5 in the above format} \\ 3y=6x-5 \\ y=(6x-5)/(3) \\ y=2x-(5)/(3) \\ \therefore By\text{ comparison, slope(m)=2} \end{gathered}`

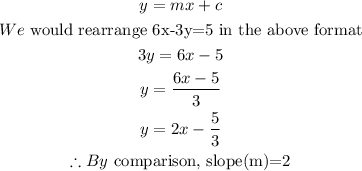
Since the line given in the questions is parallel to the required line, therefore,

`m_1=m_2`

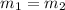
This implies their slopes are the same.

Next, we can apply all the derived parameters into the point-slope formula for the equation of a line and simplify to get the slope-intercept form

`\begin{gathered} y-y_1=m(x-x_1) \\ y-3=2(x-(-2)) \\ y-3=2(x+2) \\ y-3=2x+4 \\ y=2x+4+3 \\ y=2x+7 \end{gathered}`

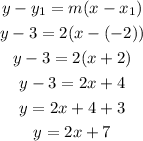
Therefore the required equation of the line is

Answer: y = 2x + 7