Question

Parallelogram ABCD is dilated to form parallelogram EFGH. Side BC is proportional to side CD. Which corresponding side is proportional to segment FG? Type the answer in the box below. (5 points)

Answer 1

The side that is proportional is GH

Hope this helps!

Answer 2

Answer with explanation:

Pre-Image=Parallelogram ABCD

⇒When ║gm ABCD is Dilated , as Preimage is larger, Dilation factor is <1,we get Image parallelogram EFGH.

Image=Parallelogram EFGH

⇒It is also given that, side BC is proportional to side CD.

→BC ∝ CD

→BC=k ×CD

`(BC)/(CD)=k`

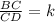
Now, the Original Parallelogram ABCD and Dilated Parallelogram EFGH will be similar,that is their corresponding sides will be proportional and corresponding interior angles will be equal.

⇒∠A=∠E, ∠B=∠F,∠C=∠G,∠D=∠H.

`\Rightarrow (AB)/(EF)=(BC)/(FG)=(CD)/(GH)=(AD)/(EH)\\\\\Rightarrow(BC)/(FG)=(CD)/(GH)\\\\\Rightarrow(BC)/(CD)=(FG)/(GH)=k`

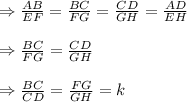
⇒Corresponding side proportional to segment F G is G H.