Given the line segment JK, point M is its midpoint, this means that it divided the line segment in two smaller segments of equal size:
JM=MK
JK= JM+MK
Since the distance from J to M is the same as the distance from M to K, first step is to calculate said distance:
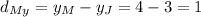
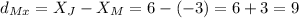
The distance between points J and M is 1 unit over the y-axis and 9 units over the x-axis, this is the same distance point K is from point M.
If you graph both points in the cartesian system and link them with a line, you'll see that point K is located in the fourth quadrant.
So to determine its coordinates, you have to add the calculated distance to the y- coordinate of M:
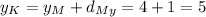
And subtract the distance over the x-axis to the x-coordinate of M
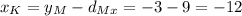
The coordinates of K are (-12,5)
The correct option is the first one.