Question

How do you find the slope of this function? And How do you know if the slope from the left and right are the same for this piecewise function?

Answer 1

The slope for the first part of the function is 1 since this is a line represented by

`f(x)=-1+x`

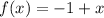
The slope of this line is 1 (it is the coefficient of x). If we take its derivative, the result is 1.

For the second part of the function, we need to take its derivative:

We have that the derivative for a constant is 0. So, the derivative for 1 is zero.

And we know that:

`(d)/(dx)(x^n)=nx^(n-1)`

So

`(d)/(dx)(-x^2)=-2x^(2-1)=-2x`

`(d)/(dx)(1-x^2_{})=-2x`

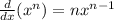
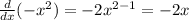
So, in this case, is a line with a slope of -2x, and then, the slopes of both piecewise function are different. In the first case, slope = 1, and in the second case, slope= -2x.

In the first function, for x near to 1, the slope is 1. For the second function (parabola) for x = 1, the slope is -2(1) = -2.