Given
To show from first principles that the derivative of a constant is zero .
Step-by-step explanation
We have to prove that from the first principle the derivative of a constant is zero.
To prove :
If f(x) =c, for some constant c, then f'(x) = 0.
Proof :
Suppose f(x) = c for some constant c.
Then the derivative of f(x) can be determined as
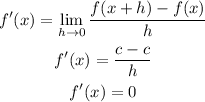
Hence proved.