Answer: Yes, ABC is a right triangle.
Explanation:
Since, a triangle is called right triangle if the square of one side is equal to the sum of the squares of other two sides.
Here, the vertices of triangle ABC are A(–2, 3), B(–3, –6), and C(2, –1).
By the distance formula,
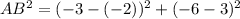
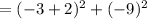
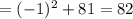
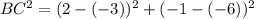
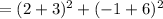
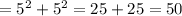
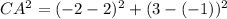
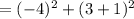
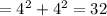
Since,
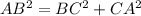
Hence, ABC is a right angled triangle.