Consider the polynomial
 .
.
For this polynomial to be trinomial with a degree of 3. We have to identify the missing exponent of the 'y' term.
Let the missing exponent of the 'y' term be 0
So, the polynomial will be
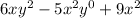
=
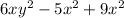
=
 which is not a trinomial, which is a binomial.
which is not a trinomial, which is a binomial.
So, the missing exponent of 'y' can'not be zero.
Let the missing exponent of the 'y' term be 2
So, the polynomial will be
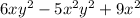
=
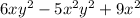
=
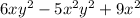 which is a trinomial of degree 4.
which is a trinomial of degree 4.
So, the missing exponent of 'y' can'not be '2'.
If we will take the missing exponent of 'y' more than 2, than the degree of the given polynomial will not be 3 anymore.
Let the missing exponent of the 'y' term be 1
So, the polynomial will be
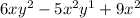
=
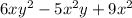
=
 which is a trinomial of degree 3
which is a trinomial of degree 3
So, the missing exponent of 'y' is 1.