Question

The value of a dirt bike decreases by 30% each year. If you purchased this dirt bike today for $500, to the nearest dollar how much would the bike be worth 5 years later?

$84
$119
$164
$222

Answer 1

it starts at $500, then decreases by 30% so just multiply 500 by .7, then multiply the answer by .7, and so on until you get to $84.

Answer 2

After 5 years cost of the dirt bike will be $84.

Option A is the answer.

Explanation:

The value of a dirt bike decreases by 30% each year. If the present cost of the dirt bike is $500.

Then the function which defines the decrease in cost of the bike will be

`f(t)=a(r)^(t)`

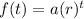
where a = $500

After one year decrease in the cost of the bike will be = 500×0.30 = $150

The cost of the bike next year will be = 500-150 = $350

So the sequence for the cost every year will be 500, 350..........

Now the common ratio of the sequence will be =
`(350)/(500)=0.70`

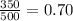
Now we can form the explicit formula for this geometric sequence as

`a_(n)=a(r)^(n)`

`a_(n)=500(0.70)^(n)` where n is the time in years.

For t = 5 years

`a_(5)=500(0.70)^(5)`

`a_(5)=500(0.168)`

`a_(5)=84.035`

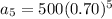
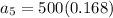
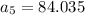
Therefore, after 5 years cost of the dirt bike will be $84.

Option A is the answer.