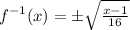Question

Which equation is the inverse of y = 16x^2 + 1?

Answer 1

Switch y and x: x =16y^2 + 1
Solve for y: x-1=16y^2
16y =+-√(x-1)
y =+-√(x-1)/16

Answer 2

The inverse of the equation is
`f^(-1)(x)=\pm\sqrt{(x-1)/(16)}`

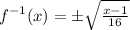
Explanation:

Given : Equation
`y=16x^2+1`

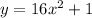
To find : The inverse of the equation ?

Solution :

Equation
`y=16x^2+1`

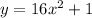
To find the inverse we interchange the value of x and y,

`x=16y^2+1`

Now, solve for y

`16y^2=x-1`

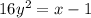
Divide by 16 both side,

`y^2=(x-1)/(16)`

Taking root both side,

`y=\pm\sqrt{(x-1)/(16)}`

`f^(-1)(x)=\pm\sqrt{(x-1)/(16)}`

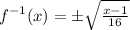
Therefore, The inverse of the equation is
`f^(-1)(x)=\pm\sqrt{(x-1)/(16)}`