Question

Given triangle ABC, A = 120°, a = 8, b = 3; determine B to the nearest whole degree. Do not use a decimal point or extra spaces in the answer or it will be marked incorrect.

Answer 1

the answer is 45
8/120. = 3/x cross multiply

Answer 2

∠B = 19°

Explanation:

In the triangle ABC,

∠A = 120° side a = 8 and b = 3

We have to find angle B.

We will apply sine rule in this triangle

`(a)/(sinA)=(b)/(sinB)`

`(8)/(sin120*)=(3)/(sinB)`

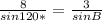
8 sin B = 3 sin 120°

Sin b =
`(3)/(8)` (sin 120°) =
`(3* 0.866)/(8)` = 0.3248

B = sin⁻¹ (0.3248)

= 18.95 ≈ 19°

Therefore, ∠B = 19° is the answer.