Question

Find a degree 4 polynomial having zeros -5, -2, 4 and 8 and the coefficient of x^4 equal 1.The polynomial is

Answer 1

The polynomial is:

`x^4-5x^3-42x^2+104x+320`

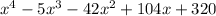
Step-by-step explanation

Note: If n is a zero of a polynomial, then (x-n) is a factor of the same polynomial. Hence, the factors for the given zeros are:

P(x) = (x+5)(x+2)(x-4)(x-8)

Let's multiply these out to obtain the polynomial in Standard Form.

`\begin{gathered} P\mleft(x\mright)=(x+5)\mleft(x+2\mright)\mleft(x-4\mright)\mleft(x-8\mright) \\ P(x)=(x^2+2x+5x+10)(x^2-8x-4x+32) \\ P(x)\text{ = }(x^2+7x+10)(x^2-12x+32) \\ P(x)=x^4-12x^3+32x^2+7x^3-84x^2+224x+10x^2-120x+320 \\ P(x)\text{ = }x^4-5x^3-42x^2+104x+320 \end{gathered}`