Let the amount of 80% fruit juice needed be "x".
Let the amount of 100% fruit juice needed be "y".
We need 36 ounces in total, so we can write:

Also,
80% of x and 100% of y would be needed to form 95% of x and y, thus we can write:
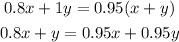
The first equation can be solved for y:
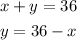
Substituting this into the second equation, we can solve for x. Shown below:
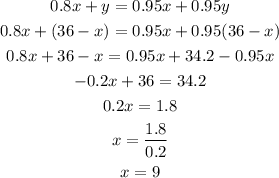
Now, we can easily solve for y:
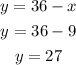
Thus, we need to combine 9 oz of 80% fruit juice with 27 oz of 100% fruit juice to get 36 oz of 95% fruit juice.