Question

For 10 years, the population of a city increases in a pattern that is approximately exponential. Using exponential modeling you find a function p(t) to describe the population where p is the population (in thousands) of the city in yeart. Your function uses an initial value of 15 and a 6.4% annual growth rate. What is a reasonable prediction for the future population of the city?

Answer 1

Answer:

The city

Explanations:

The initial population of the city, P₀ = 15000

Annual growth rate, r = 6.4% = 6.4/100

r = 0.064

An exponential function is represented by the equation:

`P(t)=P_0(1+r)^t`

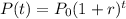
To find the population of the city after 10 years, substitute

t = 10, P₀ = 15000, and r = 0.064 into the equation above

`\begin{gathered} P(10)=15000(1+0.064)^(10) \\ P(10)=15000(1.064)^(10) \\ P(10)\text{ = }15000(1.86) \\ P(10)\text{ = }27900 \end{gathered}`

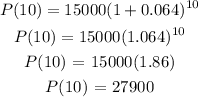
The future population of the city after 10 years is 27900