Given the triangle OPQ:
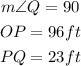
We need to find the measure of angle O
So,
As angle Q = 90, so, the hypotenuse is the side OP
The side PQ represents the opposite side to the angle O
so,
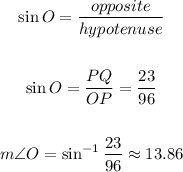
Rounding to the nearest degree:
So, the answer will be: the measure of angle O = 14