Question

F(x) is the function that determines the absolute value of the cube of the input question: determine F(5) • F(-7) number 6 part 3

Answer 1

The absolute value of cube function F(5)*F(-7) is 42,875

What is absolute value?

The absolute value of a real number is its distance from zero on the number line, regardless of the direction. It is denoted by vertical bars, like |x|, where x is the real number.

Mathematically, if x is greater than or equal to zero, then |x| is equal to x. If x is less than zero, then |x| is equal to the negation of x, making it positive.

Given

F(x) is the function that determines the absolute value of the cube of the input.

F(x) = x³

Absolute function is

`|x {}^(3) |`

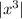
Evaluate F(5)

`F(5) = | {5}^(3) |`

`F(5) = |125|`

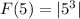
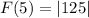
F(5) = 125 absolute value

`F( - 7) = | { - 7}^(3) |`

`f( - 7) = | - 343|`

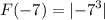
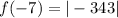
= 343 absolute value

F(5)*F(-7) = 125* 343

= 42,875

The absolute value of cube function F(5)*F(-7) is 42,875

Answer 2

We know that F(x) is the funtion that determines the absolute value of the cube of the imput, this means that F(x) is defined as:

`F(x)=\lvert x^3\rvert`

Then:

`\begin{gathered} F(5)\cdot F(-7)=\lvert5^3\rvert\cdot\lvert(-7)^3\rvert \\ =\lvert125\rvert\cdot\lvert-343\rvert \\ =125\cdot343 \\ =42875 \end{gathered}`

Therefore F(5)*F(-7)=42875.