Answer:
53.13 degrees.
Step-by-step explanation:
Given the vertices of ABC: A(4,5), B(1,1) and C (-2,5)
First, determine the side lengths AB, AC, and BC using the distance formula:
![\begin{gathered} Distance=√((x_2-x_1)^2+(y_2-y_1)^2) \\ AB=\sqrt[]{(4-1)^2+(5-1)^2}=\sqrt[]{(3)^2+(4)^2}=\sqrt[]{25}=5 \\ AC=\sqrt[]{(4-(-2))^2+(5-5)^2}=\sqrt[]{(6)^2}=6 \\ BC=\sqrt[]{(1-(-2))^2+(1-5)^2}=\sqrt[]{(3)^2+(-4)^2}=\sqrt[]{25}=5 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/dfhh4dr1xcm1nvds2b68.png)
A rough sketch of the triangle is attached below:
The base angles of the Isosceles triangle are angles A and C.
Next, we find the value of angle A using the Law of Cosine.
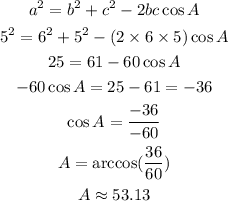
The measure of each base angle is 53.13 degrees (correct to 2 decimal places).
CHECK