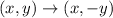Question

Identify the reflection rule on a coordinate plane that verifies that triangle A(-1,7), B(6,5), C(-2,2) and A'(-1,-7), B'(6,-5), C'(-2,-2) triangle are congruent when reflected over the x-axis.

A) (x, y) → (-x, -y)
B) (x, y) → (x, -y)
C) (x, y) → (-x, y)
D) the triangles are not congruent

Answer 1

Answer B

Step-by-step explanation:

Answer 2

Option B is correct.

The reflection rule is followed here is,
`(x,y) \rightarrow (x, -y)`.

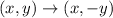Step-by-step explanation:

Since, the triangle is reflected over the x-axis , then only y- coordinates will get changed but the x coordinates will remain the same.

`A(-1,7) \rightarrow A'(-1,-7)`

`B(6,5) \rightarrow B'(6,-5)`

`C(-2,2) \rightarrow C'(-2,-2)`

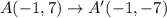
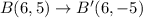
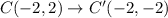
Therefore, the only reflection rule on the coordinate plane that verifies the triangle are congruent when reflected over x-axis is,
`(x,y) \rightarrow (x, -y)`