Question

Which value must be added to the expression x2 + x to make it a perfect-square trinomial?

Answer 1

x² + x + ...m

x² + x + 1/4
\/x² = x
\/(1/4) = 1/2
2*x*1/2 = 2x/2 = x

x² + x/2 + 1/4

Answer 2

For this case we have the following expression:

`x^2 + x`

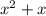
We want to find a constant to create a perfect-square trinomial

The value of the constant is given by:

`k = (\frac {b} {2}) ^ 2`

Where, b belongs to the coefficient that accompanies the term of exponent 1 in the quadratic equation.

`b = 1`

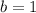
Substituting values:

`k = (\frac {1} {2}) ^ 2\\k = \frac {1} {4}`

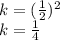
Rewriting the expression we have:

`x^2 + x + \frac {1} {4} = (x + \frac {1} {2}) (x+\frac {1} {2})\\x^2 + x + \frac {1} {4} = (x + \frac {1} {2}) ^ 2`

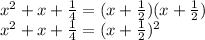
Answer:

`k = \frac {1} {4}` must be added to the expression
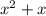`x^2 + x` to make it a perfect-square trinomial