Question

What value in place of the question mark makes the polynomial below a perfect square trinomial? 9x2+?x+64

Answer 1

9x² + bx + 64
\/(9x²) = 3x
\/84 = 8
2*3x*8 = 48x
b = 48

Answer 2

When A = 48, the polynomial
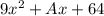`9x^2+Ax+64` a perfect square trinomial.

Explanation:

Given : the polynomial
`9x^2+Ax+64`

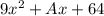
We have to find the value of A that makes the polynomial a perfect square trinomial.

For a trinomial to be a perfect square trinomial when it can be written in form of a perfect square as
`(a+b)^2=a^2+b^2+2ab`

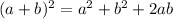
Consider the given polynomial
`9x^2+Ax+64`

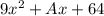
Comparing the given polynomial with the right side of the above identity,

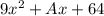`9x^2+Ax+64` can be written as
`(3x)^2+Ax+(8)^2`

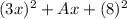
We have,

a = 3x , b = 8

also , 2ab = Ax

2(3x)(8) = Ax

⇒ 48 = A

Thus, When A = 48, the polynomial
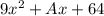`9x^2+Ax+64` a perfect square trinomial.