Question

A cubic polynomial with rational coefficients has the roots 6 + *sqrt* 6 and 2/3 . Find one additional root.

-6-*sqrt*6
6+*sqrt*6
-6+*sqrt*6
6-*sqrt*6

Answer 1

Answer:
`6 - √(6)`

Explanation:

The Conjugate Root Theorem states if P(x) is a polynomial with rational coefficients, then irrational roots of P(x) = 0 that have the form
`a + √(b)` occur in conjugate pairs. That is, if
`a + √(b)` is an irrational root with a and b rational, then
`a - √(b)` is also a root.

Since
`6 + √(6)` is a root, then
`6 - √(6)` is also a root.

Answer 2

The correct answer is 6 - sqrt(6)

We know this because when we solve with the quadratic equation, the numbers come out with a constant plus and minus the discriminant under a square root symbol. You can see that below in the quadratic equation.


`\frac{-b +/- \sqrt{ b^(2) - 4ac } }{2a}`

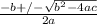
So seeing that one answer is 6 + sqrt(6), we can assume 6 is the discriminat and 6 is the constant. Thus giving us the new answer as well.