Question

Which point could be removed in order to make the relation a function? {(0, 2), (3, 8), (–4, –2), (3, –6), (–1, 8), (8, 3)} Which point could be removed in order to make the relation a function? {(0, 2), (3, 8), (–4, –2), (3, –6), (–1, 8), (8, 3)}

Answer 1

(3,-6) because you can't have two of the same x's in a function

Answer 2

ANSWER: Either
`(3,8)` or
`(3,-6)`

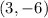
Step-by-step explanation

For a relation to be a function, it must either be a one-to-one relation or many-to-one-relation.

The above relation is one-to-many relation because 3 is mapping on to -6 and 8.

To make the above relation a function, we must remove one of the points containing 3 as first coordinate.

This will make the relation one-to-one which then becomes a function.

See attachment for diagrammatic representation.