Ok, so sec x = 1 / cos x, by using this relation you can exchange it and try to change it into something more recognisable. Let take only the left hand side only and check if the equation will be -1 at the end.
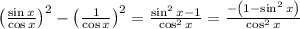
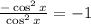
And fortunately, it is the same as the right hand side, which concludes our proof.