Given:
a varies jointly as b and c.
a=6 when b=2 and a=3.
To find:
The variation constant and the equation of variation.
Solution:
a varies jointly as b and c.

 ...(i)
...(i)
Where, k is the constant of proportionality.
a=6 when b=2 and a=3.
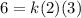

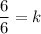
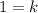
The value of k is 1.
Putting k=1 in (i), we get

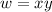
Therefore, the variation constant is 1 and the equation of variation is
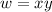 .
.