Question

Find the perimeter of the triangle whose vertices are the following, specified points in the plane.

(-10,-3), (1,4) and (-1,7)

Answer 1

Given:

The vertices of the triangle are (-10,-3), (1,4) and (-1,7).

To find:

The perimeter of the triangle.

Solution:

Distance formula:

`d=√((x_2-x_1)^2+(y_2-y_1)^2)`

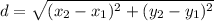
Let the vertices of the triangle are A(-10,-3), B(1,4) and C(-1,7).

Using distance formula, we get

`AB=√((1-(-10))^2+(4-(-3))^2)`

`AB=√((1+10)^2+(4+3)^2)`

`AB=√((11)^2+(7)^2)`

`AB=√(121+49)`

`AB=√(170)`

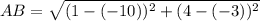
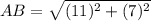
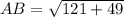
Similarly,

`BC=√(\left(-1-1\right)^2+\left(7-4\right)^2)=√(13)`

`AC=√(\left(-1-\left(-10\right)\right)^2+\left(7-\left(-3\right)\right)^2) =√(181)`

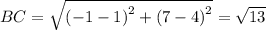
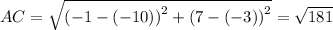
Now, the perimeter of the triangle is

`Perimeter=AB+BC+AC`

`Perimeter=√(170)+√(13)+√(181)`

`Perimeter\approx 13.038+3.606+13.454`

`Perimeter=30.098`

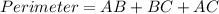
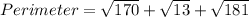
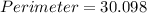
Therefore, the perimeter of the triangle is 30.098 units.