Question

It's almost time to go home. You spent a lot of money this vacation! You are counting up the money you have left. You only have dimes and quarters, and you have a total of 103 coins worth $15.25. How many dimes do you have? How many quarters do you have? Solve using Substitution.

Answer 1

From the information provided, we have a total of 103 coins. We do not know the number of dimes and quarters but its all a total of $15.25. This can be rewritten as 1525 cents.

Note also that;

`\begin{gathered} 10\text{dimes}=\text{ \$1}=100\text{cents} \\ 4\text{quarters}=\text{ \$1}=100cents \end{gathered}`

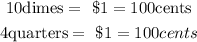
For the total number of coins, we would have;

`\begin{gathered} d+q=103---(1) \\ \end{gathered}`

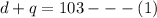
For the total amount of money available, we would have;

`10d+25q=1525---(2)`

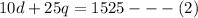
We would now take equation (1). Make d the subject of the equation and we'll have;

`d=103-q`

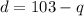
Substitute for d into equation (2);

`\begin{gathered} 10d+25q=1525 \\ 10(103-q)+25q=1525 \\ 1030-10q+25q=1525 \end{gathered}`

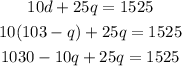
We can now collect like terms, and we'll have;

`\begin{gathered} 25q-10q=1525-1030 \\ 15q=495 \\ \end{gathered}`

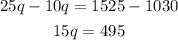
We next divide both sides by 15;

`\begin{gathered} (15q)/(15)=(495)/(15) \\ q=33 \end{gathered}`

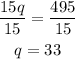
This means we have 33 quarters. We can now substitute for the value of q into equation (1);

`\begin{gathered} d+q=103 \\ d+33=103 \\ d=103-33 \\ d=70 \end{gathered}`

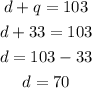
ANSWER:

We now have,

70 dimes and 33 quarters