Question

Find the indicated probabilities using the geometric distribution, Poisson distribution, or the binomial distribution. The mean number of births per minute in a given country in a recent year was about 6. Find the probability that the number of births in any given minute is exactly five. Round to four decimal places as needed.

Answer 1

0.1606 = 16.06% probability that the number of births in any given minute is exactly five.

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval.

In this question:

We only have the mean during an interval, and this is why we use the Poisson distribution.

The mean number of births per minute in a given country in a recent year was about 6.

This means that
`\mu = 6`

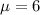
Find the probability that the number of births in any given minute is exactly five.

This is P(X = 5). So

`P(X = 5) = (e^(-6)*6^(5))/((5)!) = 0.1606`

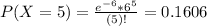
0.1606 = 16.06% probability that the number of births in any given minute is exactly five.