Question

Please help me find the answers to these questions, I don’t understand how to get through the process to then be able to answer the questions

Answer 1

Explanations:

a) Given the equation of a curve expressed as:

`y=x^3-5x^2+7x-2`

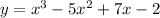
On differentiating the function, we will have:

`\begin{gathered} (dy)/(dx)=3x^(3-1)-2(5)x^(2-1)+7 \\ (dy)/(dx)=3x^2-10x+7 \end{gathered}`

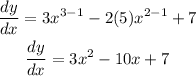
If the dy/dx = 0, then the x-ccordinates will be:

`\begin{gathered} 3x^2-10x+7=0 \\ 3x^2-3x-7x+7=0 \\ 3x(x-1)-7(x-1)=0 \\ (3x-7)(x-1)=0 \\ x=(7)/(3)and\text{ 1} \end{gathered}`

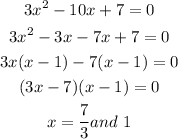
Determine the turning points

If x = 7/3 then;

`\begin{gathered} y=((7)/(3))^3^{}-5((7)/(3))^2+7((7)/(3))-2 \\ y=(343)/(27)-(245)/(9)+(49)/(3)-(2)/(1) \\ y=(343-735+441-54)/(27) \\ y=-(5)/(27) \end{gathered}`

One of the turning points is (7/3, -5/27)

if x = 1 then;

`\begin{gathered} y=x^3-5x^2+7x-2 \\ y=1^3-5(1)^2+7(1)-2 \\ y=1-5+7-2 \\ y=1 \end{gathered}`

The other turning point is at (1, 1)

b) To get the minimum and maximum point, we will find the second derivative of the function as shown:

`\begin{gathered} f^(\doubleprime)(x)=6x-10 \\ \text{If x = 7/3} \\ f^(\doubleprime)((7)/(3))=6((7)/(3))-10 \\ f^(\doubleprime)((7)/(3))=(42)/(3)-10 \\ f^(\doubleprime)((7)/(3))=(12)/(3)=4>0 \\ \end{gathered}`

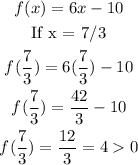
Since f''(x) > 0 at the point where x = 7/3, hence the turning point (7/3, -5/27) is at the minimum

At the point where x = 1,

`\begin{gathered} f^(\prime)(1)=6(1)-10 \\ f^(\doubleprime)(1)=6-10 \\ f^(\doubleprime)(1)=-4<0 \end{gathered}`

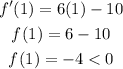
Since f''(x) > 0 at the point where x = 1, hence the turning point (1, 1) is at the maximum point.

c) To sketch the turning points (1, 1), and (7/3, -5/27)

To get other points, at x = 2

`\begin{gathered} y=2^3-5(2)^2+7(2)-2 \\ y=8-20+14-2 \\ y=0 \end{gathered}`

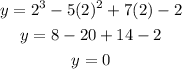
Another coordinate point will be at (2, 0)