Question

Mr. Rodriguez is packing bags of snacks for his children’s lunchboxes. If he has 20 blueberries and 30 grapes, what is the maximum number of bags of snacks that he can pack so that each bag has the same number of blueberries and grapes?

Answer 1

He can do 4 bags with 5 of each fruit.

Answer 2

The maximum number of bags is 10 with 2 blueberries and 3 grapes every one.

Explanation:

The maximum number of bags of snacks that he can pack so that each bag has the same number of blueberries and grapes is calculate finding the greatest common factor between 20 and 30.

First, it is necessary to identify all the factors of 20 and 30. Then, identify what are the factor that are equal in both cases and choose the greatest one. This is:

20 can be calculate as:

1 x 20 = 20

2 x 10 = 20

4 x 5 = 20

Then, the factors of 20 are: 1, 2, 4, 5, 10 and 20

At the same way, 30 can be calculate as:

1 x 30 = 30

2 x 15 = 30

3 x 10 = 30

5 x 6 = 30

Then, factors of 30 are: 1, 2, 3, 5, 6, 10, 15 and 30

Now, from these factors, the numbers 1, 2, 5 and 10 are factors of both numbers and the greatest one is 10. So, the greatest common factor of 20 and 30 is 10.

That means that the maximum number of bags of snacks that he can pack is 10. So, the number of blueberries that are going to be in every bag is calculate as:

`(20 Blueberries)/(10 Bags) = 2 Blueberries`

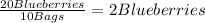
And, the number of grapes in every bag is:

`(30 Grapes)/(10 Bags) = 3 Grapes`