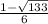Answer:
A quadratic equation
 ....[1]
....[1]
then the solution is given by:
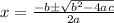
Given the equation:
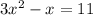
Subtract 11 from both sides we have;
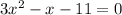
On comparing with [1] we have;
a = 3 , b =-1 and c =-11
Substitute these we have;
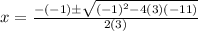
⇒
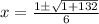
⇒
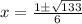
Therefore, the solution for the given equations are:
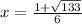 ,
,